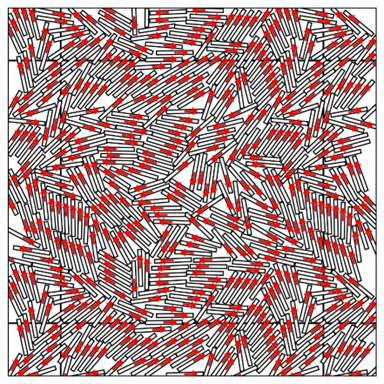
In ordered materials, deformation is understood to occur through localized deviations from an ideal crystal lattice structure. However, in amorphous systems without such a structure, it is more difficult to predict how a material will yield under an applied strain. One method that has been successful in passive systems is to look for special quasi-localized modes that minimize an approximation of the energy barrier that must be crossed in order to rearrange.

We are interested in applying this technique to active systems, where individual particles can locally consume energy to do work. In some active systems it is still possible to define an energy functional; previous work in our group by Julia Giannini has done this for a system of active particles that are attracted to a central point. In other common self-propelled particle models such as active Brownian or Ornstein-Uhlenbeck particles there is an instantaneous energy landscape that evolves over time with the rotational degrees of freedom of the self-propulsion forces. We want to generalize these quasi-localized modes in a fully non-Hamiltonian system of self-propelled rods for which there is never a well-defined energy function. This will hopefully allow us to predict the rearrangements that occur when a jammed packing of rods is subjected to global shear or thermal fluctuations.