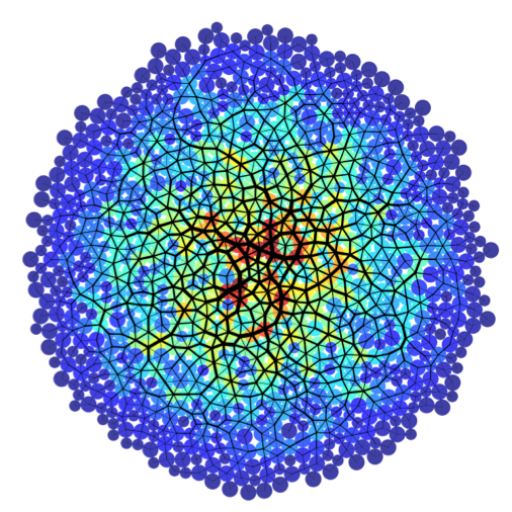
If you push a pile of sand very lightly, it will respond elastically, but if you push beyond an extremely low threshold, it will begin to flow. But the system that it flows into will be qualitatively similar to the state it was just in. We are studying that marginal transition in low dimensional systems (2D and 3D) using multiple approaches.

One approach is to use sparse random matrix models to understand the low-frequency spectrum of disordered solids. Specifically, we have found that a broad class of random matrices generate eigenvector statistics that are identical to those in the boson peak, and we are now investigating under what conditions these matrices generate the recently observed omega^4 scaling seen at the very lowest frequencies in the density of states.

Related research in disordered solids:
State changes in jammed solids
Vibrational properties of jammed particles